Nuclear physics – more than a passion, more than just a career

Professor Stuart Pittel from the Bartol Research Institute and the Department of Physics and Astronomy at the University of Delaware provides his reflections on a career in nuclear structure theory and the international collaborations that shaped it.

A major theme of my career in nuclear physics has been the search for an understanding of the collective properties exhibited by atomic nuclei and the development of the theoretical tools needed for that understanding. In what follows, I will summarise a few selected areas in which I have been able to make contributions. A common theme in all has been the key role played by international collaboration.

The search for a unified shell model description of nuclear deformation – background remarks

Beginning in the 1970s, I began to work with Pedro Federman, then at the UNAM in Mexico City, on the search for a unified microscopic understanding of how nuclear deformation arises throughout the periodic table. Early work on this subject had focused on the heavy rare earth and actinide regions, where stable deformed nuclear shapes had been known to exist for many years. The typical picture that had been used to describe the emergence of deformed equilibrium shapes in these regions involved a competition between the long-range quadrupole interaction between nucleons and the short-range pairing interaction.¹ Whereas pairing is known to favour spherical shapes, quadrupole correlations favour deformation. While pairing correlations contribute linearly as a function of valence nucleon number to the energy of the system, quadrupole correlations contribute quadratically. Thus, in nuclei with a sufficiently large number of valence nucleons, the quadrupole correlations will eventually win out and static deformed nuclear shapes will emerge.

Nuclear deformation was also known to exist in lighter nuclei, e.g. in the 2s1d shell, where both the valence neutrons and protons occupy the same major shell. In this region, the nuclear shell model had been used to study the emergence of deformation and it was shown that the strong mixing needed to produce these correlations derive from the proton-neutron interaction between particles occupying the same set of valence orbitals, with the 1d5/2 and 1d3/2 orbitals playing a particularly important role.²

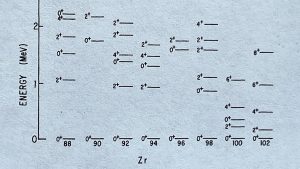
Our interest in the subject began with the discovery of an intermediate range of deformed nuclear systems in the Zr (Z = 40) and Mo (Z = 42) isotopes with neutron numbers around N = 60. These nuclei were produced in studies of the fission fragments from the spontaneous fission of 252Cf.³ The striking feature of the data is that while the Zr (see the Figure on the following page) and Mo isotopes with neutron numbers up to N=56 could be described in terms of simple shell model configurations with a minimal amount of configuration mixing and thus no collectivity, the addition of just a few more neutrons produced a very rapid onset of deformation. What made this region so attractive is that, like the lighter region where deformation was known, it was readily amenable to shell model treatment, but now with valence neutrons and protons not filling the same major shells. If we could understand how deformation arose here it might provide a bridge between the two earlier known regimes of deformed nuclei.

The picture that emerged

Our analysis showed that in this region, nuclear deformation was likewise driven by the proton-neutron interaction and arose when the correlations it produced dominated over alike-nucleon (nn and pp) pairing correlations.⁴ But what was striking is that unlike in the heavier nuclei that had been investigated to that point, it derived primarily from the interaction between neutrons and protons in selected orbits and, in particular, in the 1g9/2 proton orbit and the 1g7/2 neutron orbit rather than the cumulative involvement of many orbits. Because of the very strong attraction between protons and neutrons in these spin-orbit-partner orbitals, a large number of valence protons and valence neutrons was not needed for proton-neutron correlations to dominate pairing correlations and produce a deformed structure. Indeed, even though these were medium-mass nuclei, with a significant neutron excess, the same physics seems to underlie the rapid emergence of deformation here as in the 2s1d shell. In this way, we were able to achieve a unified picture of how deformation arises. In all regions, it derives from the domination of proton-neutron correlations (via its long-range quadrupole component) over pairing correlations. When isolated special proton and neutron orbitals, e.g. spin-orbit partners, are available, this can occur with just a few valence particles. When such isolated orbits are not available, however, it occurs slowly through the gradual build-up of pn correlations involving a much larger number of valence nucleons, the picture that was accepted at that time for the rare-earth and actinide regions of deformation.

The role of nuclear shell structure and its evolution in nuclear deformation

As should be evident from the above remarks, the relevant shell structure of the nuclei involved plays a critical role. It is primarily the interactions between valence nucleons, those outside filled major shells, that to a large extent dominate the properties of the low-lying states in nuclei and their equilibrium shapes. That this is the case can be seen from the success of the NpNn arguments put forth by Casten and collaborators.⁵ But as is now also known and the subject of much current study, this shell structure is not a static feature of nuclei but can evolve as the number of neutrons and protons change, primarily through the monopole-monopole proton-neutron interaction.⁶ Indeed, the same proton-neutron orbital pairs that produce very strong quadrupole mixing also produce rapid changes in the associated single-particle energies as they are being filled, often producing large gaps in the single-particle spectra where none previously existed.

To see how this plays out, consider the rare-earth region of deformed nuclei. Here, there are typically fairly close-lying proton and neutron single-particle levels, so that a fairly large number of valence protons and neutrons are needed to achieve deformation. As an example, in the Ce isotopes, with Z = 58 and thus eight valence protons outside a magic Z = 50 core, deformation sets in very gradually as a function of neutron number and is fairly complete by 152Ce with 12 valence neutrons outside magic N = 82. Now consider the Gd isotopes, with Z = 64. The addition of six more protons produces a rather different scenario. Here, there is a fairly large gap in the neutron single-particle spectrum near N = 90, which, as discussed by Casten and co-workers,⁷ is due to the strong monopole-monopole interaction between 1h11/2 protons and 1h9/2 neutrons. As a result, deformation in the Gd isotopes builds up fairly rapidly near N = 90, especially when contrasted with the more typical isotopic chains in the rare-earth region.

A second point to note is that while we focused on the key role of spin-orbit partner orbitals, subsequent work has shown that neutron and proton orbitals that are linked by the tensor interaction likewise have very strong interactions in both the quadrupole and monopole channels and that they too can lead to dramatic effects in producing strong evolution in the shell structure of nuclei and the rapid onset of deformation.⁸ Recent work on the study of nuclei in regions far from stability have shown many new examples of rapid evolution in shell structure due to such strong monopole-monopole effects.

Pairing correlations in nuclei

As noted earlier, pairing correlations also play a key role in nuclear structure physics and in the collective features exhibited by nuclei. They typically arise from the short-range attraction between nucleons occupying the same single-particle orbital. In the case of identical nucleons, for which the isospin of a pair is T = 1, the angular momentum and parity of a correlated pair that optimally exploits this attraction is Jπ = 0+, with orbital angular momentum L = 0. These correlations, as noted earlier, favour spherical shapes.

Since the late 1950s, it has been recognised that nuclei can exhibit a form of superconductivity⁹ due to the alike-nucleon pairing force, analogous to that familiar for electrons in solids. There, the usual framework for describing such correlations is the number-nonconserving Bardeen Cooper Schrieffer (or BCS) Theory.¹⁰ While the same theory has also been used for treating pairing correlations in nuclei, it can produce errors at some level for finite nuclear systems which have a relatively small number of active nucleons. This has been recognised for a long time, and number conserving variants of the BCS theory have been developed to overcome this limitation.¹¹

But there are also other issues regarding the BCS or even the number-conserving BCS (PBCS) approach. Both assume a condensate of collective pairs having the same structure. Recent work, in some of which I have been involved, places limitations on this picture. A theoretical tool that has made it possible to probe details of pairing correlations can be found in the early 1960s work of Richardson¹² who showed that a pure pairing hamiltonian can be solved exactly. Based on this, and in collaboration with Jorge Dukelsky at the CSIC in Madrid and Carlos Esebbag from the University of Alcala likewise in Spain, I have carried out some work that illustrates how full superconductivity in finite nuclear systems emerges gradually. As the pairing strength is increased, more and more pairs gradually become fully correlated and contribute to the superconductivity.¹³

Some of these ideas were further developed in collaboration with Jorge Dukelsky, Willy Dussel (from Buenos Aires) and Pedro Sarriguren (from the CSIC in Madrid) within the context of the Skyrme Hartree Fock approach for more realistic nuclear systems in the even Sm isotopes (Z = 62).¹⁴ We first obtained a self-consistent set of deformed mean-field single-particle states and then compared how pairing correlations emerge when applying the BCS, PBCS, and exact Richardson approaches to this mean field. There, we were able to show that while PBCS captures some of the correlations missing in the traditional BCS approach, it still misses some for nuclei away from shell closure. Most significantly, we were able to show that the collective pairs closest to the Fermi surface were the most highly correlated, and that the level of collective correlation decreases as we move further from the Fermi Surface.

Proton-neutron pairing correlations in nuclei

More recently, strong interest has developed in the role of proton-neutron pairing correlations, which can potentially play an important role in lighter nuclei where the valence neutrons and protons occupy the same major shell and especially those near N = Z. While this remains a key area of both experimental and theoretical research, there have been some important advances and I will briefly comment on one to which I contributed.

In the late 1990s, Jan Dobes from the Academy of Sciences of the Czech Republic in Prague and I carried out a study within the context of an exactly solvable model that involved both isoscalar (T = 0, S = 1) and isovector (T = 1, S = 0) pairing.¹⁵ A key conclusion of this work was that a system with all three pairing modes active, nn, pp, and np, will naturally favour quartet correlations involving two neutrons and two protons with total isospin T = 0. A simple qualitative argument for this is that the smallest object that can simultaneously reflect all three modes of pairing on an equal footing is such a 2n − 2p quartet (i.e. an alpha-like cluster). We also showed that in the vicinity of N = Z, a mean-field description that does not include such correlations tends to produce states with strongly mixed isospin character.

There have been extensive recent efforts aimed at explicitly incorporating such quartet correlations in the description of nuclei, both with N = Z and with additional alike-nucleon pairs.¹⁷ These works further demonstrate their importance and suggest their usefulness in providing a simple interpretation of such systems.

International collaborations and their impact on my work and my life

In the mid-1970s, I was contacted by Pedro Federman to begin a collaboration. At the time, Federman was at the UNAM in Mexico City, but he was originally from Buenos Aires. We had collaborated earlier while I was a postdoctoral researcher at the Bartol Research Foundation, now the Bartol Research Institute, and he was interested in continuing our work together. This began what has been for me an incredible journey through a series of international collaborations that has lasted until today. From my collaborations with Federman to a career-long collaboration with Jorge Dukelsky, initially from Buenos Aires and eventually from Madrid to collaborations with Mario Stoitsov from Sofia, Piet Van Isacker from GANIL in Caen, Roelof Bijker, Jorge Hirsch, and Alejandro Frank from the UNAM, Nicu Sandulescu from Bucharest, YuMin Zhao from Shanghai, Lei Yang from Mianyang, and so many others too numerous to elaborate, this has been essential to my research career.

Before a trip to visit one of my collaborators, my brother-in-law commented: “Stu travels to distant lands to speak with the only other two people in the world that have the foggiest idea of what he’s talking about.” Though said tongue-in-cheek, it in fact has a small grain of truth to it. Nuclear physics, and my particular area of Nuclear Structure Theory, is indeed a small community, with many of the leading institutions and centres of study being located outside my home country, the United States. With English being the international language of science, it is relatively easy to build and foster international scientific collaborations, and this can be of enormous benefit to the resulting science, as it most certainly has been for me.

But at the personal level, my collaborations have been about so much more than the science alone. Lengthy visits to work with my collaborators, either me visiting them or them visiting me, have also had an incredible impact on my personal life. Many of the scientists with whom I have worked have also become among my closest friends, with their families becoming mine and vice versa. This has helped make nuclear physics so much more than just a scientific career for me. It embodies a community and it has truly shaped my life!

Dedication and acknowledgments

This article is dedicated to the memory of two of my most valued collaborators and dearest friends, Pedro Federman and Mario Stoitsov, both of whom passed away much too early. I would also like to acknowledge Rick Casten and Jorge Dukelsky for helpful suggestions about the manuscript.

References

1. B. R. Mottelson, in Proceedings of the International School of Physics, ‘Enrico Fermi’ Course XV, Varenna, 1960, ed. G. Racah, Academic, New York, 1962, p. 44
2. I. Talmi, Rev. Mod. Phys. 34 (1962) 704; I. Unna, Phys. Rev. 132 (1963) 2225
3. E. Cheifetz, R. C. Jared, S. G. Thompson and J. B. Wilhelmy, Phys. Rev. Lett. 25 (1970) 38
4. P. Federman and S. Pittel, Phys. Lett. 69B (1977) 385; P. Federman and S. Pittel, Phys. Lett. 77B (1978) 29; P. Federman, S. Pittel and R. Campos, Phys. Lett. 82B (1970)9; P. Federman and S. Pittel, Phys. Rev. C20 (1979) 820
5. See e.g. R. F. Casten, Nucl. Phys. A443 (1985) 1
6. See e.g. E. Caurier, G. Martinez-Pinedo, F. Nowacki, A. Poves and A. P. Zuker, Rev. Mod. Phys. 77 (2005) 427 and references therein
7. R. F. Casten, D. D. Warner, D. S. Brenner and R. L. Gill, Phys. Rev. Lett. 47 (1981) 1433
8. T. Otsuka, R. Fujimoto, Y. Utsuno, B.A. Brown, M. Honma and T. Mizusaki, Phys. Rev. Lett. 87 (2001) 082502; T. Otsuka, T. Suzuki, R. Fujimoto, H. Grawe and Y. Akaishi, Phys. Rev. Lett. 95 (2005) 232502
9. A. Bohr, B. R. Mottelson and D. Pines, Phys. Rev. 110 (1958) 936
10. J. Bardeen, L. N. Cooper and J. R. Schrieffer, Phys. Rev. 108 (1957) 1175
11. K. Dietrich, H. J. Mang and J. H. Pradal, Phys. Rev. 135 (194) B22
12. R. W. Richardson, Phys. Rev. Lett. 3 (1963) 277; Phys. Rev. 141 (1966) 949
13. J. Dukelsky, C. Esebbag and S. Pittel, Phys. Rev. Lett. 88 (2002) 062501
14. G.G. Dussel, S. Pittel, J. Dukelsky and P. Sarriguren, Phys. Rev. C76 (2007) 011302(R)
15. J. Dobes and S. Pittel, Phys. Rev. C57 (1998) 688
16. See e.g. N. Sanduelscu, D. Negrea, J. Dukelsky and C. W. Johnson, Phys. Rev. C85 (2012) 061303R; N. Sandulescu, D. Negrea and D. Gambacurta, Phys. Lett. B751 (2015) 348; N. Sandulescu and M. Sambataro, Phys. Rev. C93 (2016)054320
17. See e.g. D. Negrea, P. Buganu and N. Sandulescu, Phys. Rev. C98 (2018) 064319

Professor Stuart Pittel
The Bartol Research Institute
Department of Physics and Astronomy
University of Delaware
+1 302 831 1103
pittel@udel.edu
Tweet @UDphysics
www.bartol.udel.edu/~pittel/Welcome.html

Please note, this article will also appear in the seventh edition of our quarterly publication.